Risk factors of ventilator-induced lung injury: mechanical power as surrogate of energy dissipation

Soon after physicians began to artificially ventilate patients with respiratory insufficiency due to poliomyelitis (1) and intensive care units developed, risks of mechanical ventilation—namely ventilator-induced lung injury (VILI)—became evident (2). The recognition of this iatrogenic, and potentially lethal, syndrome led to a slow change in the goal of mechanical ventilation for respiratory failure: from maintaining near-normal gas exchange, with use of large tidal volumes and high airway pressures (3), to avoiding additional lung damage—the so-called “lung protective” ventilation (4). The last 60 years of preclinical and clinical research in the field focused on identifying the single ventilator variable most responsible for VILI, with no conclusive answer. For instance, seminal animal studies showed that very large tidal volumes (“volutrauma”) and/or very high peak airway pressures (“barotrauma”) lead to overt pulmonary edema in previously healthy animals (5). More recently, high inspiratory flows and high respiratory rates emerged as other risk factors for lung damage (6,7).

The “mechanical power” is defined as the energy delivered every minute to the lungs, or the respiratory system as a surrogate, during mechanical ventilation. It is computed as energy per cycle multiplied by respiratory rate and is expressed in joules per minute (J/min) (8). On a dynamic pressure-volume curve recorded during tidal ventilation, the energy delivered per cycle to the respiratory system (including the artificial airway) corresponds to the area between the inspiratory limb of the airway pressure and the volume axis. Alternatively, it can be readily and quite accurately computed from volume, pressure and flow displayed on common multi-parametric monitors, according to a modified equation of motion, as shown below. The mechanical power thus depends on, and summarizes, all the variables currently implicated in the pathogenesis of VILI: volume, pressure, flow and respiratory rate. Therefore, it may have a higher predictive value for VILI than any of its individual components.

In line with this premise, a study recently published in Intensive Care Medicine examined the association between mechanical power and in-hospital mortality in critically-ill patients receiving mechanical ventilation for at least 48 hours (9). Serpa Neto and coworkers retrospectively analyzed the data of 8,207 patients stored in two large databases, the MIMIC-III and the eICU, referred to 59 hospitals in the USA, from 2001 and 2015. Most of the patients in both databases were admitted to Intensive Care from the emergency department, with a medical diagnosis; only 10.6% of them had the Acute Respiratory Distress Syndrome (ARDS) at admission. Mechanical power was independently associated with in-hospital mortality in either the MIMIC-III (odds ratio per 5 J/min increase 1.06; 95% confidence interval 1.01–1.11; P=0.021) or the eICU (1.10; 1.02–1.18; P=0.010). This finding remained valid even when the analysis was restricted to patients ventilated with low tidal volume (≤7.5 mL/kg predicted body weight) or low driving pressure (≤13 cmH₂O), as part of a lung protective ventilation strategy, and to those without ARDS. The risk of death generally started to increase when mechanical power exceeded 17.0 J/min. These data suggest that mechanical power provides additional information for the clinician to recognize potentially harmful ventilator settings.

In the next few sections we will address some key issues related to the mechanical power: (I) its meaning and relevance for the pathogenesis of VILI; (II) how to compute it at the bedside; (III) its major limitations.

From lung viscoelasticity to energy dissipation

In response to an external force, a perfectly elastic material undergoes deformation (“strain”) and develops an internal tension (“stress”). As soon as the force is removed, it returns to its original, stress-free, shape. In other words, all energy accumulated during loading is returned during unloading. Similarly, when the mechanical ventilator generates a transient positive pressure gradient at the airway opening, lungs inflate and then passively deflate. However, for any given lung volume, the pressure needed for inflation is always higher than the one recorded during exhalation (hysteresis of the pressure-volume loop). Only some of the energy (pressure times volume) delivered during inflation by the mechanical ventilator is recovered from the lungs by the end of the cycle, while a part is irreversibly dissipated. This phenomenon is typical of viscoelastic, non-perfectly elastic, materials such as the lungs.

But where does unrecovered energy go? Energy is a conserved quantity: it cannot just “disappear”. If it is not returned, it must have been transferred somewhere to perform some work. During mechanical ventilation, some energy is lost to generate the gas flow against airway resistance, to expand the chest wall, to overcome the superficial tension of the alveolar liquid-air interface. Some other energy is dissipated to unfold the intricate network of elastin fibers, collagen fibers and ground substance forming the lung fibrous skeleton, that is to win the internal viscous resistances against the structural rearrangement required for lung inflation (10). If excessive, this energy may disrupt the microstructure of the fibrous skeleton, trigger inflammation (11) and thus initiate or propagate VILI.

Unfortunately, the amount of energy delivered to the lung fibrous skeleton during inflation and not returned during exhalation cannot be measured at the bedside. Nevertheless, one can expect a positive relationship between energy delivery and energy dissipation (12). As long as the mechanical power is related to the energy delivered to the respiratory system, it will also reflect the amount of dissipated energy possibly implicated in the pathogenesis of VILI.

Mechanical power equation

To fully understand the concept of the mechanical power (and its equation), it is helpful to consider the classical equation of motion [1]:

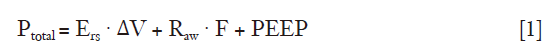
Where:

P_total: Total pressure of the respiratory system at any given time.

E_rs ∙ ΔV: Elastance of the respiratory system times the tidal volume. This term can be easily simplified by obtaining the pressure (P_{tidal elastic}) required to inflate the tidal volume into the respiratory system (i.e., the driving pressure).

R_aw ∙ F: Resistances of the airways times the flow, i.e., the pressure needed to move the gas through the airways.

PEEP: Positive end-expiratory pressure. This is the pressure needed at end-expiration to keep the volume of the respiratory system above functional residual capacity (FRC).

Thus, it is possible to describe and quantify the total pressure generated by the ventilator in order to move gas inside the respiratory system during a single breath as:

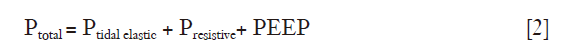
If we now consider a closed system with a defined volume, applying a pressure to the system will result in a change in volume. This variation of volume (ΔV) that the system undergoes, multiplied by the pressure achieved, yields to energy in joules delivered to the system. This physical concept can be applied to the respiratory system as well, as graphically shown in Figure 1. The inspiratory (red) and expiratory (green) pressure-volume curves of a single breath are represented. The energy delivered during inspiration is the area between the inflation red line and the y-axis, while the energy delivered to the respiratory system but not recovered during expiration (respiratory system hysteresis) is the area between the inflation (red) and deflation (green) lines.

Figure 1 Mechanical power components. Schematic representation of the inspiratory (red arrows) and expiratory (green arrows) pressure-volume curve of a single breath. The area between the inflation line and the y-axis represents the energy delivered during inspiration, while this energy multiplied by the respiratory rate is the mechanical power. The area between the inflation and deflation lines represents energy delivered to the respiratory system but not recovered during expiration (respiratory system hysteresis). Of note, both mechanical power and respiratory system hysteresis overestimate energy dissipated at the lung tissue level, which is the one possibly implicated in the pathogenesis of VILI (see text). Elastic component (A): the area of the triangle with plateau airway pressure and tidal volume as base and height, respectively; Resistance to airflow component (B): the area of the vertically shaded parallelogram with (peak—plateau) airway pressure as base and tidal volume as height; Viscous resistance component (C): the area of the blue squared parallelogram with (P₁—plateau) airway pressure as base and tidal volume as height; PEEP component (D): the area of the dotted rectangle with PEEP as base and tidal volume as height. Note that the energy required to inflate PEEP into the system, i.e., the area of the white triangle with PEEP as base and PEEP volume as height, is required only once and thus is not part of the mechanical power equation. ΔV, tidal volume; P_plat, plateau airway pressure; P_peak, peak airway pressure; P₁, post-occlusion airway pressure; PEEP, positive end-expiratory pressure.

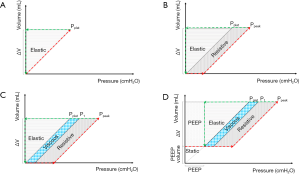
The product of the total pressure (determined by the three different components listed above) and the tidal volume is the amount of energy needed to inflate the lungs and the chest-wall:

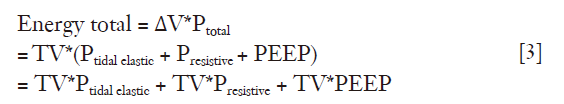
Each component of the formula is an “energy pack” delivered to the respiratory system per breath. Adding the respiratory rate to the formula [3] we obtain the complete mechanical power equation (in joules/minute) with its four components:

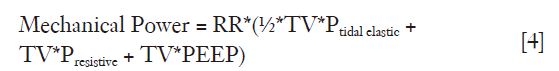
Where:

TV*P_resistive is the energy required to move gas.

½*TV*P_{tidal elastic} is the energy required to inflate the lung and chest wall from their initial position; note that this term is divided by 2 due to the triangular shape of the elastic component of the energy delivered per breath (Figure 1).

TV*PEEP is the energy required to overcome positive end-expiratory pressure (PEEP)—related recoil of the respiratory system.

RR is the respiratory rate (the number of cycles per minute).

The mechanical power equation [4], can be rearranged in several ways. Indeed, as P_{tidal elastic} is equal to P_plat – PEEP and P_resistive is equal to P_peak − P_plat, substituting these values into [4] results in the following formula, that was used by Serpa Neto and coworkers themselves:

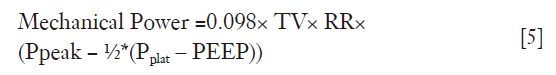
Mechanical power as a risk factor for VILI: limitations

Some limitations of mechanical power need to be mentioned. First, in physics “power” is the time-derivative, or the rate, of the energy yielded to the system (during inspiration) or by the system (during expiration). By contrast, mechanical power reflects the cumulative energy delivered to the respiratory system over a period of time, with multiple breathing cycles. They are both expressed in joules/min but they convey different information. A similar difference exists between inspiratory flow (time derivative of tidal volume; analogue to power) and minute ventilation (tidal volume times respiratory rate; mechanical power). A single large breath of 1 litre delivered in one second with a respiratory rate of 5 breaths per minute will yield a quite large inspiratory flow (1 litre/second) but a normal minute ventilation (5 litres/minute). Again, both variables have the same units of measure, but they convey different information. For a given mechanical power, delivering large amounts of energy in a very short time frame (e.g., a single breath) could damage the lungs even if the total energy delivered in a minute is low, due to a low respiratory rate. Being provocative, one single inflation above total lung capacity could produce gross barotrauma, even if the cumulative energy delivered in a minute is not large.

Second, factors other than mechanical power reasonably contribute to harms of mechanical ventilation, including the condition of the aerated lungs (for instance, density and severity of in-homogeneities acting as energy amplifiers) and extra-pulmonary variables (including hemodynamics).

Third, mechanical power is an imprecise measure of the energy dissipated at the lung tissue level. For instance, considering peak airway pressure in the calculation of power clearly overestimates the energy delivered to the lungs, since most of that pressure is spent to overcome airways resistances, the highest fraction of which is represented by the endotracheal tube. In other words, tracheal pressure will always be much lower than peak airway pressure, and this difference will increase with decreasing endotracheal tube size. This issue hinders the comparison of mechanical power between different patients. The energy dissipated outside the lung and the energy recovered by the end the respiratory cycle should not contribute to VILI. A way to “unmask” dissipated energy at the alveolar level is to keep volume constant during an end-inspiratory pause and to measure pressure, which will decrease over time approaching a plateau value (plateau airway pressure). This phenomenon, called “stress relaxation”, can be quantified as slow post-occlusion pressure decay, from a point immediately following peak airway pressure (P₁) to the plateau pressure (P_plat or P₂) (13). Preliminary findings from our laboratory suggest that high P₁–P₂ following an end-inspiratory occlusion, possibly reflecting large energy dissipation during ongoing mechanical ventilation, is associated with the risk of developing VILI (6).

Conclusions

Mechanical power combines, in a single variable, several potential determinants of VILI: volume, pressure, flow and respiratory rate. It can be easily computed at the bedside, using commonly available data. Pending some deeper understanding of its strengths and limitations, it is easy to imagine that mechanical power will be automatically displayed on any ICU mechanical ventilator in the near future, as a guide for lung protective ventilation.

Acknowledgements

None.

Footnote

Conflicts of Interest: The authors have no conflicts of interest to declare.

References

1. Lassen HC. A preliminary report on the 1952 epidemic of poliomyelitis in Copenhagen with special reference to the treatment of acute respiratory insufficiency. Lancet 1953;1:37-41. [Crossref] [PubMed]
2. Webb HH, Tierney DF. Experimental pulmonary edema due to intermittent positive pressure ventilation with high inflation pressures. Protection by positive end-expiratory pressure. Am Rev Respir Dis 1974;110:556-65. [PubMed]
3. Pontoppidan H, Geffin B, Lowenstein E. Acute respiratory failure in the adult. N Engl J Med 1972;287:690-8. [Crossref] [PubMed]
4. Acute Respiratory Distress Syndrome Network, Brower RG, Matthay MA, et al. Ventilation with lower tidal volumes as compared with traditional tidal volumes for acute lung injury and the acute respiratory distress syndrome. The Acute Respiratory Distress Syndrome Network. N Engl J Med 2000;342:1301-8. [Crossref] [PubMed]
5. Dreyfuss D, Saumon G. Ventilator-induced lung injury: lessons from experimental studies. Am J Respir Crit Care Med 1998;157:294-323. [Crossref] [PubMed]
6. Protti A, Maraffi T, Milesi M, et al. Role of strain rate in the pathogenesis of ventilator-induced lung edema. Crit Care Med 2016;44:e838-45. [Crossref] [PubMed]
7. Cressoni M, Gotti M, Chiurazzi C, et al. Mechanical power and development of ventilator-induced lung injury. Anesthesiology 2016;124:1100-8. [Crossref] [PubMed]
8. Gattinoni L, Tonetti T, Cressoni M, et al. Ventilator-related causes of lung injury: the mechanical power. Int Care Med 2016;42:1567-75. [Crossref] [PubMed]
9. Serpa Neto A, Deliberato RO, Johnson AEW, et al. Mechanical power of ventilation is associated with mortality in critically ill patients: an analysis of patients in two observational cohorts. Int Care Med 2018;44:1914-22. [Crossref] [PubMed]
10. Protti A, Votta E. Role of tissue viscoelasticity in the pathogenesis of ventilator-induced lung injury. In: Vincent JL. eds. Annual update in intensive care and emergency medicine 2018, vol. 47. 2nd ed., Cham: Springer International Publishing; 2018, pp. 193-204.
11. Jiang D, Liang J, Fan J, et al. Regulation of lung injury and repair by Toll-like receptors and hyaluronan. Nat Med 2005;11:1173-9. [Crossref] [PubMed]
12. Suki B, Stamenović D. Lung parenchymal mechanics. Hoboken, NJ, USA: John Wiley & Sons, Inc; 2011.
13. Santini A, Votta E, Protti A, et al. Driving airway pressure: should we use a static measure to describe a dynamic phenomenon? Intensive Care Med 2017;43:1544-5. [Crossref] [PubMed]